このページにある内容は，こちらのスライド(会員向け)でわかり易く説明しています．

※PC環境なら全画面表示でより見やすく，よりわかりやすい！

高校数学[総目次]

数学Ⅱ　第4章　三角関数

  スライド ノート
1. 一般角と弧度法 [無料]  
2. 一般角の三角関数 [無料]  
3. 三角関数の性質 [無料]  
4. 三角関数のグラフ [会員]  
5. 三角関数の加法定理 [会員]  
6. 三角関数の種々の公式 [会員]  
7. 三角関数の合成 [会員]  
8. 三角関数の応用 [会員]  

7.0　三角関数の合成とは

　三角関数の合成公式と呼ばれるものがある．$\sin$ と $\cos$ の1次式を $\sin$ だけ，あるいは $\cos$ だけで書き表すものである．これは要するに $\sin$，$\cos$ の加法定理で，右辺から左辺への書き換えのことである．

　三角関数が合成できる形は $a,b$ を実数の定数として

\[a\sin\theta+b\cos\theta\]

であって，

三角関数の合成ができる型

  • $\sin,\cos$ の1次式．
  • $\sin,\cos$ の中身 $(\theta)$ は同じ．
  • $\sin,\cos$ の係数 $a,b$ は正負いかなる数でもよい．

というのが特徴である．

コラム　どうして三角関数を合成するのか？

　$a\sin\theta+b\cos\theta$ と表された三角関数の式を合成する動機にはどのようなものがあるだろうか．多いのは，この形の式がとりうる値の範囲を調べたいときである．例えば $0\leqq\theta<2\pi$ に対して

\[\sin\theta+\sqrt3\cos\theta\]

のとりうる値の範囲を調べるとしよう．式の中に $\theta$ が2か所あり，それらが同じ値をとりながら変化していくわけであるが，$\sin\theta$ や $\cos\theta$ はそれぞれ値が大きくなったり小さくなったりを繰り返す関数なので，とりうる値の範囲が捉えにくい．ところが三角関数の合成公式によって

\[\sin\theta+\sqrt3\cos\theta=2\sin\left(\theta+\frac\pi3\right)\]

と書き換えてしまうと，$\theta$ が1か所になっている．するとあとは $\sin\left(\theta+\dfrac\pi3\right)$ の変化の様子を考えるだけでよくなり，式のとりうる値の範囲がわかるという訳である．2つの $\theta$ を同時に動かして考えるのは難しいが，1つだと考えやすいのである．

7.1　sin での合成

　$\sin$ の加法定理である \[\sin(\alpha+\beta)=\sin\alpha\cos\beta+\cos\alpha\sin\beta\] において，右辺から左辺に書き換えるのが $\sin$ での合成である．

例　$\sqrt3\sin\theta+\cos\theta=\mbox{□}\sin(\theta+\triangle)$

\[\begin{align*} (\mbox{左辺})&=\sqrt{(\sqrt3)^2+1^2}\left(\underline{\sin\theta\cdot\frac{\sqrt3}2+\cos\theta\cdot\frac12}\right)\\[5pt] &=2\,\underline{\sin\left(\theta+\frac\pi6\right)} \end{align*}\] 　　 ※　　　の部分で $\sin$ の加法定理を用いた．

　一般に \[\begin{align*} a\,&\sin\theta\!+\!b\cos\theta\\[5pt] &=\sqrt{a^2\!+\!b^2}\left(\!\sin\theta\!\cdot\!\frac a{\sqrt{a^2\!+\!b^2}}\!+\!\cos\theta\!\cdot\!\frac b{\sqrt{a^2\!+\!b^2}}\!\right)\\[5pt] &=\sqrt{a^2+b^2}\sin(\theta+\alpha) \end{align*}\] 　ただし $\alpha$ は， \[\cos\alpha=\frac a{\sqrt{a^2+b^2}},\ \sin\alpha=\frac b{\sqrt{a^2+b^2}}\] となる角である．

まとめ\begin{gather*} a\sin\theta+b\cos\theta=\sqrt{a^2+b^2}\sin(\theta+\alpha)\\ \mbox{ただし},\ \sin\alpha=\frac b{\sqrt{a^2+b^2}}, \cos\alpha=\frac a{\sqrt{a^2+b^2}}\end{gather*}

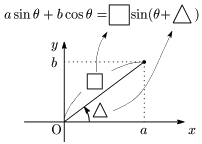
覚え方

　座標平面上に，$\sin$ の係数を $x$ 座標に，$\cos$ の係数を $y$ 座標にもつ点 $(a,b)$ をとり，原点Oと結ぶ．この線分の長さ(図の□)が合成したときの $\sin$ の係数であり，線分と $x$ 軸の正の向きとのなす角(図の△)が合成したときに用いる角である．

例題　次の式を $r\sin(\theta+\alpha)$ の形に変形せよ．ただし，$r>0$，$-\pi<\alpha<\pi$ とする．
(1)　$-\sqrt3\sin\theta+\cos\theta$
(2)　$-\sqrt3\sin\theta-\cos\theta$
(3)　$\sqrt3\sin\theta-\cos\theta$

　解答例を表示する

7.2　cos での合成

　$\cos$ の加法定理である \[\cos(\alpha-\beta)=\cos\alpha\cos\beta+\sin\alpha\sin\beta\] において，右辺から左辺に書き換えるのが $\cos$ での合成である．

例　$\sqrt3\sin\theta+\cos\theta=\mbox{□}\cos(\theta-\triangle)$

\[\begin{align*} (\mbox{左辺})&=\cos\theta+\sqrt3\sin\theta\\[5pt] &=\sqrt{1^2+(\sqrt3)^2}\left(\underline{\cos\theta\cdot\frac12+\cos\theta\cdot\frac{\sqrt3}2}\right)\\[5pt] &=2\,\underline{\cos\left(\theta-\frac\pi3\right)} \end{align*}\] 　　 ※　　　の部分で $\cos$ の加法定理を用いた．

　一般に \[\begin{align*} a\,&\sin\theta\!+\!b\cos\theta\\[5pt] &=b\cos\theta+a\sin\theta\\[5pt] &=\sqrt{b^2\!+\!a^2}\left(\!\cos\theta\!\cdot\!\frac b{\sqrt{a^2\!+\!b^2}}\!+\!\sin\theta\!\cdot\!\frac a{\sqrt{a^2\!+\!b^2}}\!\right)\\[5pt] &=\sqrt{a^2+b^2}\cos(\theta-\beta) \end{align*}\] 　ただし $\beta$ は， \[\cos\beta=\frac b{\sqrt{a^2+b^2}},\ \sin\beta=\frac a{\sqrt{a^2+b^2}}\] となる角である．

\begin{gather*} a\sin\theta+b\cos\theta=\sqrt{a^2+b^2}\cos(\theta-\beta)\\ \mbox{ただし},\ \sin\beta=\frac a{\sqrt{a^2+b^2}}, \cos\beta=\frac b{\sqrt{a^2+b^2}}\end{gather*}

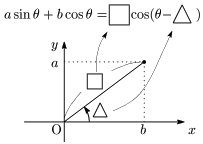
覚え方

　座標平面上に，$\cos$ の係数を $x$ 座標に，$\sin$ の係数を $y$ 座標にもつ点 $(b,a)$ をとり，原点Oと結ぶ．この線分の長さ(図の□)が合成したときの $\cos$ の係数であり，線分と $x$ 軸の正の向きとのなす角(図の△)が合成したときに用いる角である．

例題　次の式を $r\cos(\theta-\alpha)$ の形に変形せよ．ただし，$r>0$，$-\pi<\alpha<\pi$ とする．
(1)　$-\sqrt3\sin\theta+\cos\theta$
(2)　$-\sqrt3\sin\theta-\cos\theta$
(3)　$\sqrt3\sin\theta-\cos\theta$

　解答例を表示する

このページで疑問は解決されましたか？

　こちら から数学に関するご質問・ご要望をお寄せください。

高校数学[総目次]

数学Ⅱ　第4章　三角関数

  スライド ノート
1. 一般角と弧度法 [無料]  
2. 一般角の三角関数 [無料]  
3. 三角関数の性質 [無料]  
4. 三角関数のグラフ [会員]  
5. 三角関数の加法定理 [会員]  
6. 三角関数の種々の公式 [会員]  
7. 三角関数の合成 [会員]  
8. 三角関数の応用 [会員]